Dolph-Chebyshev Example

In the previous page on Dolph Weights, the Dolph-Tschebysheff method was introduced. On this page, we'll run through an example. Consider a N=6 element array, with a sidelobe level to be 30 dB down from the main beam (S=31.6223). We'll assume the array has half-wavelength spacing, and recall that the Dolph-Chebyshev method requires uniform spacing and the array to be steered towards broadside (and yes, everyone spells Chebyshef in a different way, which is why I keep changing the spelling). 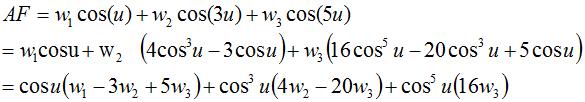The array has an even number of elements, so we'll write the array factor as: Using the trigonometric identities for the cosine function, the above equation can be rewritten as: We'll calculate our parameter (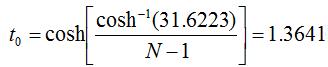) as: Then we'll substitute for cos(u) into the last equation for the AF as described previously: 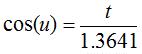We now have a polynomial of order N-1 = 5, so we'll use the Chebyshef polynomial T5(t), and equate that to our new array factor: 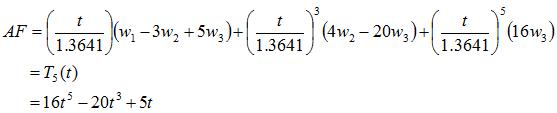The above equation is valid for all values of t. Hence, the terms that multiply t must equal, the terms that multiply t cubed must be equal, and the terms that multiply t to the 5th power must be equal. As a result, we have 3 equations and 3 unknowns, and we can easily solve for the weights: 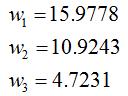The resulting normalized AF is plotted in Figure 1. 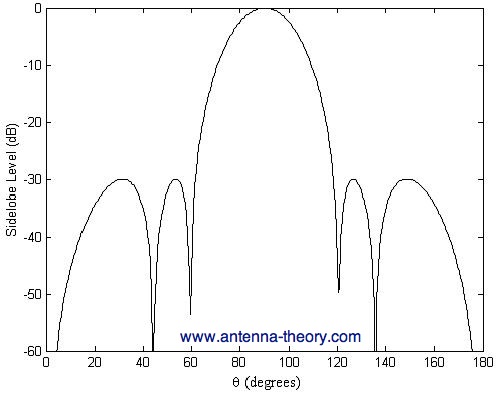Figure 1. Normalized array factor for the example on this page. Note that as desired, the sidelobes are equal in magnitude and 30 dB down from the peak of the main beam. The beamwidth obtained here (approximately 60 degrees) is the minimum possible beamwidth obtainable for the specified sidelobe level using any weighting scheme. This is the Dolph-Tschebysheff method. The basic math is all used in designing digital filters that have equal-ripple filter characteristics in the pass and stop bands. Note that if you understand digital signal processing, weight selection in antenna arrays is simple. And if you've learned something about weighting methods in antenna arrays, you've learned something about designing filters for digital systems. The underlying mathematics is largely the same.