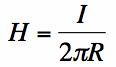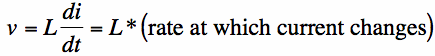The Magnetic Field
| Electric Charge | Electric Field | Magnetic Field | Antennas (Home) |
| Lenz's Law | Inductance | Capacitance | E-Field Conditions |
| H-Field Conditions |
|
As seen on the previous page, the Electric Field arises due to electric charges. It doesn't matter how the electric charges got there, be it a voltage source across a capcitor or someone rubbing a glass rod with silk (that does produce a net charge on the rod), the only thing that matters is that charge exists, and gives rise to an electric field. The world of electromagnetics has another gem waiting to be discovered. Suppose the electric charges are moving. The flow of electric charge is known as electric current. Well, electric current produces a Magnetic Field (H-field). Unlike the Electric Field which points towards or away from electric charge, the magnetic field circles a flow of charge. This is illustrated in the Figure 1:
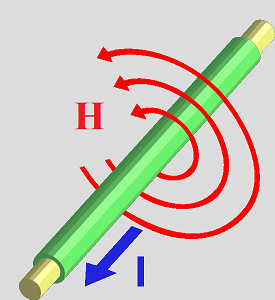 Figure 1. The Magnetic Field (H-Field) Wraps Around a Flowing Electric Current. Electric Current is the flow of charge, measured in Amps and typically written I. One Amp is equal to 1 Coulomb/second. If I Amps are flowing through a straight wire, the magnetic field that surrounds the wire a distance R from the wire is given by:
Equation [1] states that the magnitude of the magnetic field decreases with distance as 1/R from the wire. The Magnetic field is also directly proportional to the current I. Figure 1 above tells us that the direction of the magnetic field is determined by the right hand rule: if the thumb of your right hand points in the direction of the current flow, your fingers wrapping around the wire determine the direction of the magnetic field. The Magnetic field is a vector quantity like the Electric Field. The magnitude is given by equation [1] and the direction doesn't point away, towards, or in the same direction of the wire, but wraps around the wire. The units for the Magnetic Field are Amps/meter [A/m]. Lenz's Law
Imagine a single loop of wire, without current flowing through it. In the center of the loop, their will exist an upward directed Magnetic Field (think right hand rule - current flows in the same direction around the loop, and the magnetic field wraps around the current, which is always pointed upwards in the center of the loop). See Figure 2:
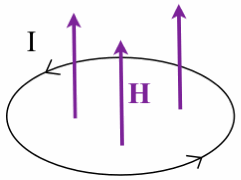 Figure 2. Current Flowing Around a Loop Produces a Magnetic Field within the Loop.
Now, electromagnetics is all about duality. So if a current flowing in a loop produces a magnetic field, what if there exists a magnetic field and we place a loop of wire in the field? It turns out, as soon as the magnetic field is applied, there will be an induced current that acts to oppose this new magnetic field. This is known as Lenz's Law. Note that this induced current only occurs when the magnetic field within the loop is changing. So let's look at a quick example. Suppose the wire loop is sitting with no external magnetic field at time t=0. Then from t=0 to t=1 the magnetic field is linearly increasing, let's say to 2 Amps/m [A/m]. Then from t=1 and on the Magnetic Field is constant. The resulting induced current will resemble that as shown:
 Figure 3. An Externally Applied Magnetic Field Changing as Shown on the Left-Hand Figure. The Induced Current is shown on the Figure on the right.
In Figure 3, I drew the induced current as negative to indicate it acts to oppose the changing magnetic field. Note that the induced current only exists when the magnetic field is changing. This is an experimentally found result. The take-away point is: nature doesn't like the magnetic field within a loop (when averaged over the area of the loop is known as magnetic flux) changing, and will produce an electric current to oppose this change. Inductance
Now that we know what a Magnetic Field is, we can define inductance. If you ask someone with a degree in physics, they will go back to their texts and start talking about flux density and turns of a coil, and at the end of the day you won't know anything except a bunch of equations. So what is inductance? A classic inductor is a looped coil: 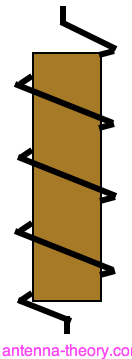 Figure 4. A classic inductor as a looped wire around some material.
A looped coil with current flowing through it produces it's own magnetic field within the coil. The field in the coil represents stored energy. So when the current source is shut off, nature will produce an opposite current that counteracts the decreased current (and hence the lossed magnetic field within the coil). The result is that a time changing electric current (i) travelling through an inductor must produce a net voltage across the inductor, which is given by:
The proportionality constant, L, is known as the inductance. This is a measure of how tightly the wires wrap around themselves, how many turns the coil has (N), the material within the inductor, etc. So - inductance is a measure of resistance to change in electric current. It is associated with stored energy in the magnetic field. Here are parameters that affect the inductance:
And that is about all you need to know about inductance to have a working knowledge of the subject.
Next: Capacitance Top: Magnetic Fields Topics Related To Antenna Theory Antennas Tutorial (Home)
|