Wave Vector
|
The Wave Vector (or wavevector) refers to a vector that describes the phase variation of a plane wave, in 3-orthogonal directions (x, y, and z-axes typically). The magnitude of the wavevector is the wavenumber.
For a wave propagation in a direction described by the spherical coordinates
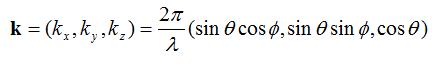
The x-component of the wavevector,
The wave vector is a property of plane waves. The phase variation for a plane wave will always be
Hence, the magnitude of the wave vector will be equal to the wavenumber. Therefore:
If
Antenna Tutorial (Home)
This page on the wave vector is copyrighted. No portion can be reproducted except by permission from the author. |
,
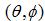the wavevector k is given by:
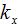, determines the rate of change of the phase
of a propagating plane wave in the +x-direction. The same definitions apply for the y- and
z-directions.
.
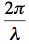